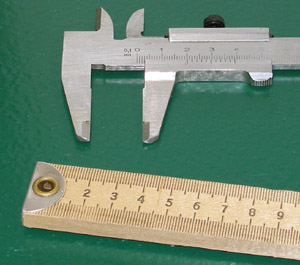
They differ in in precision.

|
| These are tools for measuring
distance.
They differ in in precision. |
Measurement plays a central role in science. Qualitative observation (that can be expressed in terms of words, rather than numbers) is also important, but many of the fundamental laws of nature can only be stated in quantitative terms. For example, "Energy is conserved" is a statement that a certain number remains constant; this has meaning only if the number can be measured.
However, relying on measurement brings its own problems. Successive measurements of "the same" quantity give slightly different results because there are always uncontrolled variables: a slight puff of wind, a vibration due to a door slamming somewhere, or the positions of the sun and moon. For example, the stop watch is precise to a hundredth of a second, but when dropping a coin, we found that measurements of the same thing could differ by several tenths of a second. Sometimes errors of this sort are referred to as "human error," which blames the discrepancy on the operator of the stopwatch, when the real fault is in the expectation that people can start and stop the watch to such precision. With better watches and better ways to start and stop them the variability could be decreased, but it cannot be eliminated.
There are two different ways to characterize the performance of the stopwatch (or any other measuring instrument). The precision tells us how finely it can divide time; the accuracy tells us how well a measurement agrees with other measurements, using the same watch or a different one. Measurement goes beyond giving numerical values for things; we also need to know how believable the values are. If two measurements differ, it does not mean that one of them is wrong. Owing to the limitations of the measuring equipment and our ability to use them, a certain amount of variability in the measurements is inevitable; subsequent measurements are expected to differ accordingly. Understanding how to deal with this is a basic scientific skill. Being able to distinguish between real differences (between physically distinct outcomes) and measurements that differ only due to the experimental variability will be important in understanding the activities to come.
Repeating a measurement several times tells us how reproducible it is. For example, suppose we dropped a coin 2 meters. Successive trials might give the following results:
| trial | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|
| time | 0.68 sec | 0.66 sec | 0.69 sec | 0.66 sec | 0.66 sec | 0.68 sec | 0.63 sec |
Here are several definitions that are useful in describing this data:
When we measure a distance we can use a meter stick or a foot ruler. The same distance will have a different numerical representation, depending on this choice. For example, if you measure the foot ruler with the meter stick, you will find it is 0.305 meters long. To avoid saying silly things like "1 = 0.305" we should always include the units in the statement: 1 foot = 0.305 meters. When we do calculations with numbers of this sort, the result acquires its units by the laws of algebra: for example, we found above that a coin falls 2 meters in 0.66 seconds; then we can calculate the average speed
average speed = distance traveled/time elapsed = 2 m/0.66 sec
= 3.0 m/sec
Discussion of the unit on timing