 |
 |
Pendulum
What determines how long it takes for a pendulum to swing?
Materials
 |
 |
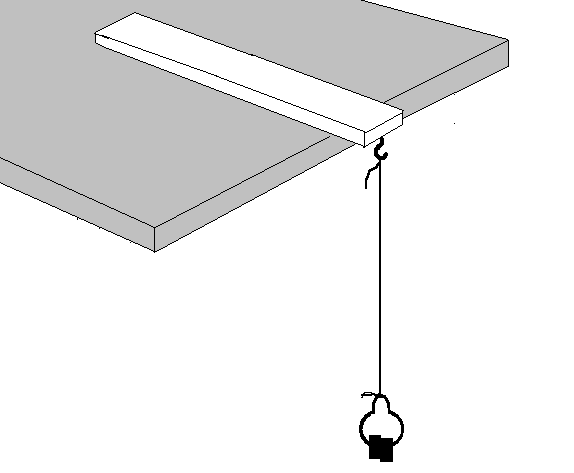
Explore a pendulum. Make one from a piece of string, a ring clip, and a few magnets. Lay the board a little over the edge of the table in a place where the pendulum can swing freely without hitting anything.
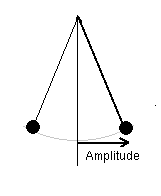
The "period" of the pendulum is how long it takes the clip with the magnets on it ("the bob") to make one complete swing back and forth, ending up where it started.
THE "displacement" is some measure of how far the bob is from the vertical position -- the horizontal distance, for example.
The "amplitude" is the maximum displacement: it is how far the pendulum goes away from the vertical position.
1. The period of the pendulum could depend (in different ways) on the mass of the bob (which can be adjusted by changing the number of magnets on the clip), the length of the string, and the amplitude. Test these variables to see what effect each one has. Make big changes (like putting four magnets on the clip). Only change one variable at a time. Describe your results for each variable.
2. In one period the pendulum travels a distance that is four times the
amplitude, and so the average speed is four times the amplitude divided by the
period. Based on your previous experience with moving systems, how would
you expect changing the mass to change the average velocity? Discuss
this both from the point of view of the Law of Force and Acceleration and
in terms of the interchange of kinetic and potential energy.

3. Making the string longer changes the path of the pendulum, so that it
is less steeply sloped. What effect would you expect this to have on the
average velocity?
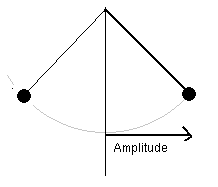
4. Making the amplitude larger gives the pendulum farther to go, but also takes it to a part of its path that is more steeply sloped? How would each change affect the period of the pendulum?
5. Design and build a pendulum that has a period of 1 second, to within 0.1
second. Describe this pendulum.
Check the box when you are done: